Essential Trigonometry Formulas All List – Solved Examples
Trigonometry Formulas provide a way to solve problems involving angles, sides, and triangles. Find angles, lengths, & areas of a triangle and solve trigon problems

What Are Trigonometry Formulas?
Trigonometry is the branch of mathematics that studies the relationship and difference between the sides and angles of triangles. It is used in many branches of mathematics, including calculus and engineering, and has applications in many fields, including astronomy, navigation, physics, and architecture.
The basic formulas of trigonometry are used to calculate the lengths of the sides and angles of a triangle. These formulas include the Pythagorean theorem, the law of sines, and the law of cosines. The Pythagorean theorem states that the square of one side of a right-angled triangle is equal to the sum of the squares of the other two sides.
The law of sines states that for all three sides of a triangle, the ratio of the length of one side to the sine of the angle opposite it is the same. And all three angles of a triangle measure 180 degrees. The law of cosines states that the square of one side is equal to the sum of the squares of the other two sides, which is twice the product of those two sides multiplied by the cosine of the included angle.
Trigonometry MCQ Mock Test Online Free
[ays_quiz id=’19’]
Trigonometric Formulas Table
| Angles (In Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
| Angles (In Radians) | 0 | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π |
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
| cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
| cosec | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
| sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1 |
Let us now know these different types of formulas in trigonometry maths:
What Are Different Trigonometric Formulas?
1. Basic Trigonometric Function Formulas
The basic trigonometric functions are sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc). They all use angles to calculate the length of two sides of a right triangle.
Example:
For example, the formula for the sine of an angle is sin(x) = opposite side ÷ hypotenuse. In a right triangle, if the angle is 30°, the opposite side is 5, and the hypotenuse is 10, then:
sin(30) = 5 ÷ 10 = 0.5
Also, read this: What Is The Formula For a2 b2, a²+b² and a²-b²? Proof & Q/A
2. Reciprocal Identities
Reciprocal identities are formulas that are used to solve trigonometric equations. They are based on the fact that the inverse of a trigonometric function is equal to the inverse of the same function. For example, the reciprocal of sine is equal to the reciprocal of sine, which is an inverse sine. This means that the mutual identity formula for sine is:
sin^-1(x) = 1/csc(x)
This means that if you know the value of x, you can use this formula to calculate the reciprocal of the sine of x.
3. Trigonometry Table
Trigonometry Table formulae are used to calculate the values of trigonometric functions given an angle in degrees or radians. A trigonometry table contains values of the trigonometric functions sine, cosine, and tangent for angles between 0° and 90°.
For example, the trigonometry table below shows the values of the sine, cosine, and tangent for angles between 0° and 90°.
Angle Sine Cosine Tangent
0° 0 1 0
30° 0.5 0.866 0.577
45° 0.707 0.707 1
60° 0.866 0.5 1.73
90° 1 0 undefined
4. Periodic Identities
Periodic identities in trigonometry are mathematical formulas that express the cyclic nature of a trigonometric function.
For example, the cosine function has a period of 2π radians, which means that it repeats its values every 2π radians. This can be expressed as an identity: cos(x+2π) = cos(x). This identity can be used to simplify calculations since it allows us to manipulate an expression without changing its value.
5. Co-function Identities
Co-function identities are formulas that relate trigonometric functions of complementary angles. For example, the co-function identity for sines and cosines states that sin(90° – θ) = cos θ. This means that the sine of the complement of any angle is equal to the cosine of that angle.
6. Sum and Difference Identities
Sum and difference identities are equations in trigonometry that express the sum and difference of two angles in terms of the trigonometric functions of those angles.
For example, the sum identity for sine is:
sin(α + β) = sinαcosβ + cosαsinβ
This equation states that the sine of the sum of two angles (α + β) is equal to the sine of the first angle (α) multiplied by the cosine of the second angle (β), plus the cosine of the first angle (α). Multiply by the sine of the other angle (β).
7. Double Angle Identities
Double angle identities are mathematical equations used to simplify some trigonometric expressions. They involve the double of a given angle and are used to solve problems such as finding the exact value of a trigonometric function for a given angle.
For example, the double angle identity for sine is given as:
sin2𝜃 = 2sin𝜃cos𝜃
This equation can be used to find the value of sine for a given angle, 𝜃:
sin2𝜃 = 2sin3θ°cos3θ°
= 2(1/2)(√3/2)
= √3/2
8. Triple Angle Identities
Triple angle identities are formulas that allow you to express a trigonometric function of multiple angles in terms of trigonometric functions of simpler angles.
For example, the triple angle identity for the cosine function is:
cos(3x) = 4cos³(x) – 3cos(x)
This identity allows us to express the cosine of 3x in terms of the cosine of x.
9. Half Angle Identities
Half-angle identities are mathematical equations that express trigonometric functions of half angles in terms of trigonometric functions of the original angle. For example, the identity of half an angle for cosine is
cos(θ/2) = √((1 + cosθ) /2).
This equation can be used to find the cosine of the half angle by first finding the cosine of the original angle and then plugging it into the equation.
10. Product Identities
Product identities in trigonometry are mathematical equations that relate the three sides of a triangle (or the three angles) to one another. For example, the Pythagorean Theorem is a product identity that states that the sum of the squares of the sides of a right triangle is equal to the square of the hypotenuse. In other words, a2 + b2 = c2.
11. Sum to Product Identities
Sum to Product Identities are equations that are used to convert the sum of two trigonometric functions into the product of two trigonometric functions. For example, the equation for converting the sum of two sines into a product of two sines is:
sin(A) + sin(B) = 2sin( (A + B)/2 ) * cos( (A – B)/2 )
Also, read these:
- 10 Root Vegetables Name | जमीन के अंदर तैयार होने वाली 10 सब्जियां
- 6 Flower Vegetables Name | फूलों वाली 6 सब्जी के नाम
- 150+ Vegetables Name in English and Hindi with Images
- 30+ Fruits Name in English and Hindi | फलों के नाम
12. Inverse Trigonometry Formulas
Inverse trigonometric functions are used to find an angle from a given ratio of two sides in a right triangle. For example, the inverse of the sine function sin-1(x) is used to find an angle θ in a right triangle when the ratio of the opposite side to the hypotenuse is known.
In this example, the angle θ is found by using the inverse of the sine function:
sin-1(x) = θ
where x is the ratio of the opposite side of the triangle to the hypotenuse.
For example, if the ratio of the opposite side of a right triangle to the hypotenuse is 0.5, then the angle θ can be found using the inverse of the sine function as follows:
sin-1(0.5) = θ = 30°
Trigonometric Formulas Table Chart or Image
Trigonometry Formulas Identities
You have already seen some trigonometric identities. It is convenient to have a summary of them for reference. These identities mostly refer to a single angle denoted by θ, but there are some that involve two angles, and for those, the two angles are denoted α and β.
The more important identities
Sine and cosine relations are used to define tangents, cotangents, secants, and cosecants.

Pythagorean formulas for sines and cosines. Trigonometric identities are probably the most important.

Identities expressing trigonometric functions in terms of their complements. These aren’t very interesting. At the complementary angle, each of the six trig functions equals its co-function.

Periodicity of trig functions. Sine, cosine, secant, and cosecant have period 2π while tangent and cotangent have period π.

Identities for negative angles. Sine, tangent, cotangent, and cosecant are odd functions while cosine and secant are even functions.

Ptolemy’s identities, the sum and difference formulas for sine and cosine.

Double angle formulas for sine and cosine. Note that there are three forms for the double angle formula for cosine. You only need to know one, but be able to derive the other two from the Pythagorean formula.

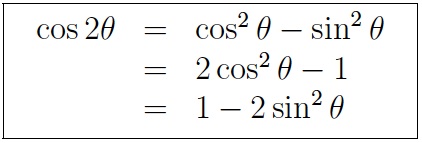
Trigonometry Formulas Involving Reciprocal Identities
1. sin (x) = 1/cosec (x)
2. cos (x) = 1/sec (x)
3. tan (x) = 1/cot (x)
4. cosec (x) = 1/sin (x)
5. sec (x) = 1/cos (x)
6. cot (x) = 1/tan (x)
Easy Steps to Learn Trigonometry Formulas
1. Understand The Basics:
Before attempting any trigonometric formula, it is important to understand basic concepts such as complementary angles, the definition of hypotenuse and sine, cosine, and tangent.
2. Memorize Common Formulas:
Once the basics are understood, it is important to memorize the most common trigonometry formulas. These include the Pythagorean theorem, the law of sines, and the law of cosines.
3. Practice Calculations:
Practice is the key to understanding trigonometry formulas. Use the practice problems to get comfortable with the formulas and understand how to apply them.
4. Use Visual Aids:
Visual aids can be very helpful in understanding trigonometry formulas. Draw diagrams that help explain the formulas and how they work.
5. Use Online Resources:
There are many online resources that can help in understanding trigonometry formulas. Watch videos and tutorials that explain formulas in easy-to-understand terms.
Real-Life Trigonometry Examples
Applications of Trigonometry:
Example 1: A man is observing a tree of height 57 feet. According to his measurement, the tree cast a 26 feet long shadow. Can you help him in determining the sun’s angle of elevation from the shadow’s tip?
Solution: Let z be the angle of elevation of the sun, then
tan z = 57/26 = 2.1923
z = tan-1(2.1923)
or z = 65.480 degrees
FAQs
Q.1: Why are trigonometric functions used?
Ans: Trigonometric functions are used to find the value of an unknown side or angle of a 90-degree triangle.
Q.2: Name the basic trigonometric functions.
Ans: Tangent, cosine, and sine are the three basic trigonometric functions.
Q.3: What are the values of sin3θ and tan3θ?
Ans: The value of sin3θ is ½, and the value of tan3θ is 1/√3.
Q.4: Who established the Pythagoras Theorem?
Ans: Greek philosopher and mathematician Pythagoras is the founder of the Pythagoras Theorem.
Q.5: What can trigonometric functions be used for?
Ans: Trigonometric functions can be used to find the height of a building, the distance between 2 vertical points, and many other practical applications.
Q.6: Where is trigonometry used in real life?
Ans: Many real-life situations are developing around trigonometric functions, such as measuring sea level every time the tide changes, and measuring the length of each day when the seasons change. Trigonometry is also mainly used to determine directions like north-south or east-west, this will help you decide which direction you should fix the compass to go in a straight line . It is used by airlines in the navigation of routes and to indicate a location in sea areas. It is also used to find the proper distance from any point in the sea to the shore.
Also, Read These Posts:
- 20 Ka Pahada | Multiplication Table Of Twenty
- Table of 19 | Multiplication Table of Nineteen
- Multiplication Times Table of Eighteen | 18 Ka Phada | अठरा का पहाड़ा
- Multiplication Times Table of Seventeen | 17 Ka Phada | सत्रह का पहाड़ा
- Multiplication Table of Sixteen | 16 Ka Phada | सोलह का पहाड़ा
- Multiplication Table of Fifteen | 15 Ka Phada | 15 का पहाड़ा
- Multiplication Times Table of Fourteen | 14 Ka Pahada | 14 का पहाड़ा
- Multiplication Table of Thirteen | 13 Ka Pahada | 13 का पहाड़ा
- Multiplication Table of Twelve | 12 Ka Pahada | Learn 12 का पहाड़ा
- Multiplication Table of Eleven | 11 Ka Pahada | 11 का पहाड़ा
- Multiplication Table of Ten | 10 का पहाड़ा | Das Ka Pahada
- Multiplication Tables of Eight | Table of 8 | 8 Ka Phada
- Multiplication Tables of 7 | 7 का पहाड़ा | 7 Ka Pahada
- Multiplication Table of Six | 6 ka Phada | Learn Table of 6
- Multiplication Tables of 5 | 5 का पहाड़ा | Panch Ka Pahad
- 4 का पहाड़ा | 4 Ka Pahada | Multiplication Table 4
- Table Of 3 – Learn 3 Ka Pahada
- Table Of 2 – Learn 2 Ka Phada